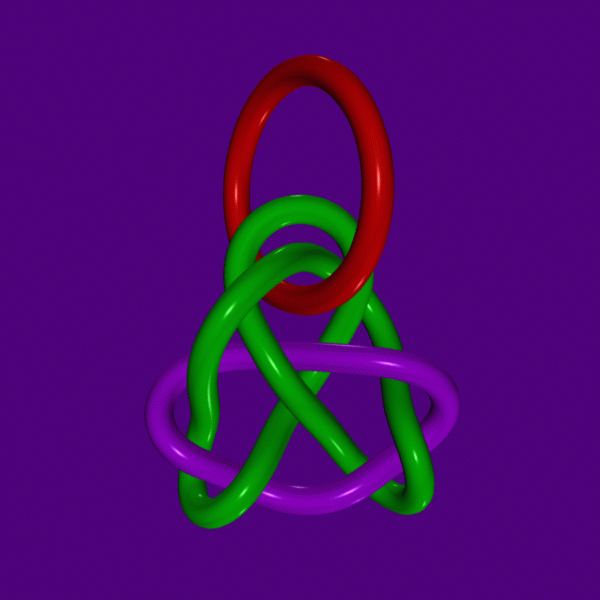The Borromean Rings

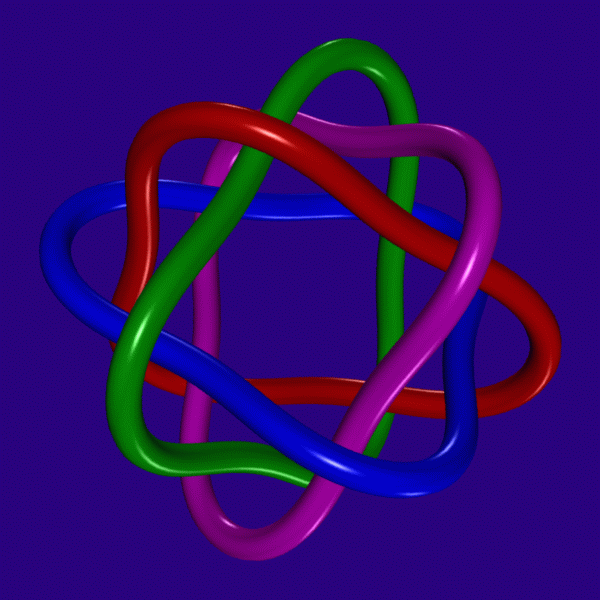
A picture of a 12-crossing alternating link, displaying a symmetry of order 4. The complement of this link may be formed by glueing together two ideal cube-octahedra.



These two cells then constitute the canonical cell decomposition of the link. The symmetry group of the link is Z₂ x P, where P is the group of rotational symmetries of a cube (equivalently of an octahedron.)

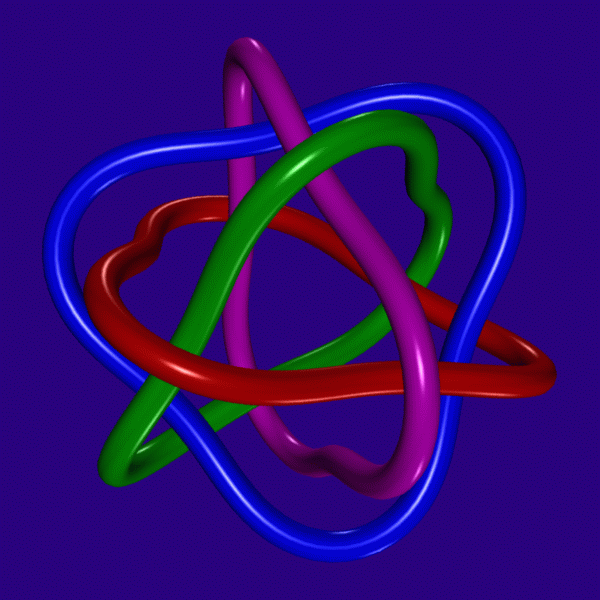
The same alternating link, displaying this time a symmetry of order 3

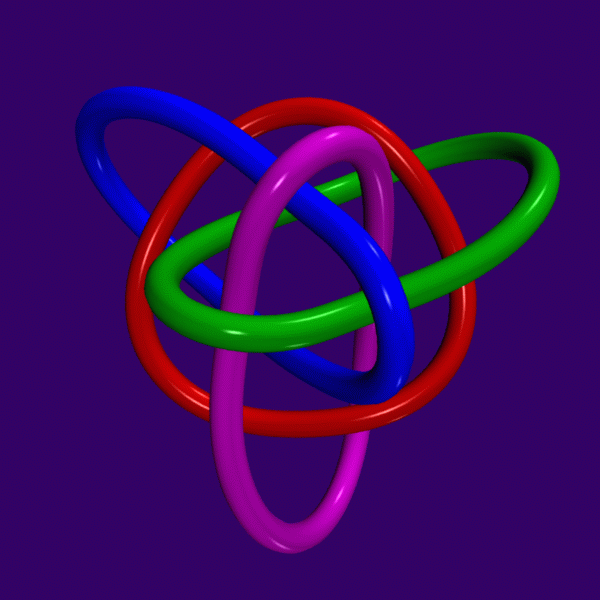
A 12-crossing non-alternating link whose canonical cell decomposition consists of a single ideal cube-octahedron.

The symmetry group of this link is the dihedral group D₃.

However, the complement of the link has extra symmetries that don't extend to the (S³, link) pair. The symmetry group of the complement is isomorphic to the group P mentioned above, and acts transitively on the four cusps.

Craig Hodgson has shown that the complement of this link is double-covered by the complement of the alternating link shown above.

For alternating hyperbolic links it seems that the symmetry group of the link complement is always equal to that of the (S³, link) pair, but this has not been proved.

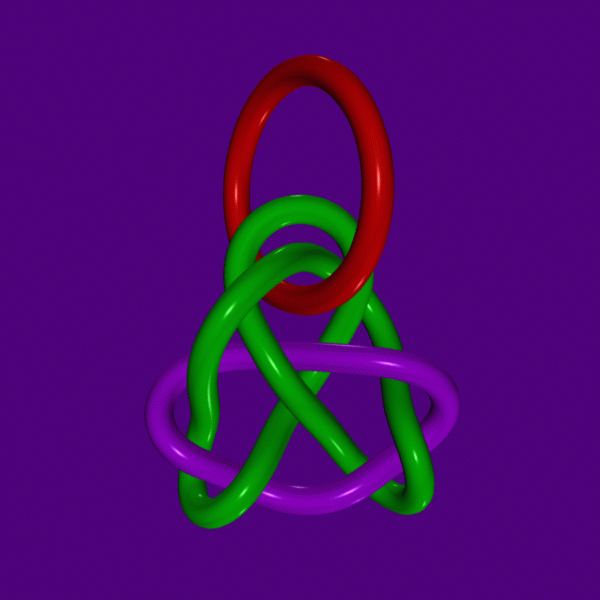
A 15-crossing non-alternating link whose canonical cell decomposition also consists of a single ideal cube-octahedron. Here the symmetry group of both link and manifold is the Klein group (of order 4.)