Periods and fixed-point-free symmetries
The purpose of this page is to illustrate the two kinds of
orientation-preserving symmetries of knots. Symmetries may be
classified according to the nature of their fixed-point sets.
Traditionally a symmetry whose fixed-point set is a circle in
S3 is called a period . We can think of a period
of order n as a rotation through 2pi/n about an axis.
Energy minimization algorithms are particularly good at picking up and
displaying this kind of symmetry. The program Knotscape , available
on this site, has an option for computing the nature of the fixed point set
of each symmetry of a hyperbolic knot.
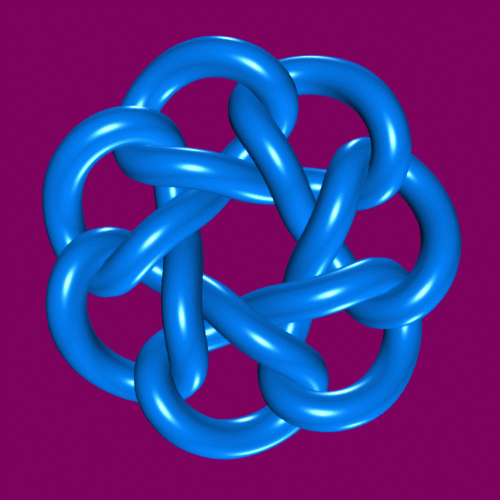
This knot has symmetry group D7;
this minimal energy presentation clearly reveals a period of order 7.
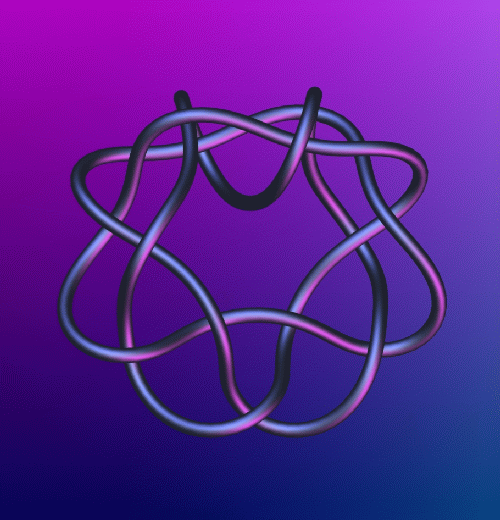
This knot also has symmetry group D7, but here the symmetries of
order 7 are fixed-point-free, hence are not rotations about an axis as
in the previous example. They are screw motions along a torus, and therefore cannot
be realized as Euclidean motions. Since all the standard energy minimization routines
are based on the Euclidean metric, it is not surprising that they do not display
such symmetries. However, it is not hard to see from this picture that the knot is
the closure of the 3-string braid (s2-1s1)7.t,
where s1, s2 are standard braid generators and t is a full twist.
Therefore we can take a free symmetry of order 7 to be
s2-1s1 combined with a meridional rotation through
2pi/7.
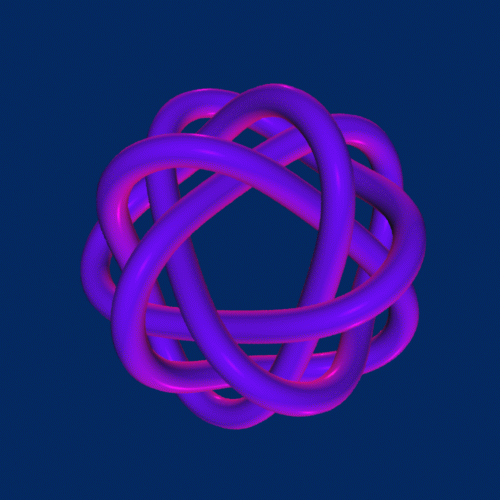
A non-alternating 18-crossing knot with a period of order 5

This picture of the 16-crossing knot with symmetry group D9
was created synthetically, in order to exhibit the fixed-point-free symmetry
of order 9. A close inspection of the picture reveals that the cube of this
fixed-point-free symmetry is a period of order 3! Therefore the cyclic subgroup
of order 9 contains both kinds of symmetry.




